Notice that the y-intercept of the line in the graph is 5. Set x=0 on the given equations to find which of them satisfy that condition. It turns out that there are two possibilities:
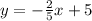

Furthermore, we can see that when x=2, then y=0 on the graph. substitute x=2 on those equations to find which of them satisfy that condition:


Then, the equation:

is the only one which matches the line on the image.