It is given that z varies directly with x and inversely with y.
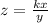
Where k is the constant of proportionality.
First, let us find the value of constant (k).
Substitute x = 6, y = 2, and z = 15
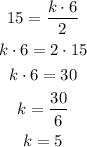
So, the value of k is 5
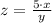
Finally, let us find the value of z when x = 4 and y = 9
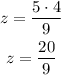
Therefore, the value of z is 20/9