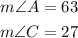You know that the triangle given in the exercise is a Right Triangle, because it has an angle that measures 90 degrees.
Knowing two sides of the triangle, you can use the following Inverse Trigonometric Function to find the measure of the angle A:
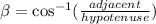
In this case:
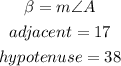
Then, substituting values and evaluating, you get:
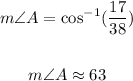
Now you can use the following Inverse Trigonometric Function to find the measure of the angle C:
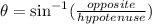
In this case:
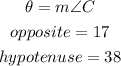
Substituting values and evaluating, you get:
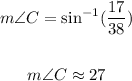
The answer is: