In a trpezium, the median line is always parallel to the bases. The expression for the length of the median of trapezium is :
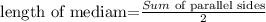
In the trapezium: NM = ML=LK=PQ=QR=RS
we have : NP = 8, LR =20
Conisder the case of trapezium NPLR, where MQ is the median
From the expression of the length of median:

Now, consider the case of trapezium MQSK, where LR is act as median, MQ= 14, LR =20
From the expression of the length of median

So, the diameter of the bottom KS is 26 inches
Answer : the diameter of the bottom KS is 26 inches