Question

Which of the following piecewise functions best describes the graph below ?The answer

Answer 1

To answer this question, we need to be careful with the domain (x values) and the slope of the lines.

From left to right we have the following functions:

For values less than or equal to -3, we have a line with a positive slope. We have two lines, y = x - 2, and y = x - 3.

If we make:

x = -3, and y = -5, then we have:

1. For y = x - 2

`-5=-3-2\Rightarrow-5=-5`

2. For y = x - 3

`-5=-3-3\Rightarrow-5\\e-6`

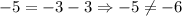
Then, we have that the second option is a possible candidate to solve the question.

We can also see that the first option is not an option since the domain is given for x less than -3 (and the graph is for x less or equal to -3 - see the solid circle of the line).

We also have that for the interval from x greater than -3 and less or equal to 5, the value for the function is equal to 8.

We also have that the line has a negative slope for values greater than 6, and the given equation is y = -x + 10.

Therefore, the answer to this question is the second option: