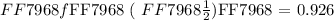We are given a function f ( x ) defined as follows:
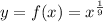
We are to determine the value of f ( x ) when,
In such cases, we plug in/substitue the given value of x into the expressed function f ( x ) as follows:
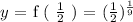
We will apply the power on both numerator and denominator as follows:
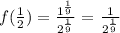
Now we evaluate ( 2 ) raised to the power of ( 1 / 9 ).
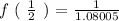
Next apply the division operation as follows:
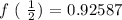
Once, we have evaluated the answer in decimal form ( 5 decimal places ). We will round off the answer to nearest thousandths.
Rounding off to nearest thousandth means we consider the thousandth decimal place ( 3rd ). Then we have the choice of either truncating the decimal places ( 4th and onwards ). The truncation only occurs when (4th decimal place) is < 5.
However, since the (4th decimal place) = 8 > 5. Then we add ( 1 ) to the 3rd decimal place and truncate the rest of the decimal places i.e ( 4th and onwards ).
The answer to f ( 1 / 2 ) to the nearest thousandth would be: