Question

PRE-CALC! The graph of y= f(x) is transformed to y= f(x-p)+q to move the graph three units left and three units down find the value of P and Q.

Answer 1

You know that the graph of:

`y=f\mleft(x\mright)`

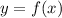
is transformed in this form:

`y=f\mleft(x-p\mright)+q`

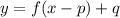
And it is moved 3 units left and 3 units down.

Then, you need to remember the following Transformation Rules for Functions:

1. When:

`f(x+h)`

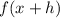
The function is shifted "h" units to the left.

2. When:

`f(x-k)`

The function is shifted "k" units down.

Then, knowing these rules, you know that the value of "p" must be negative, in order to get a positive sign when you substitute it into:

`y=f\mleft(x-p\mright)+q`

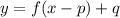
And the value of "q" must be negative.

Therefore, you get:

`\begin{gathered} y=f\mleft(x-(-3)\mright)+(-3) \\ y=f(x+3)-3 \end{gathered}`

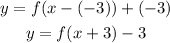
Hence, the answer is: Second option.