Let's start with the first triangle from the left side. Let's label the sides of the triangle with respect to the angle.
As we can see, the given sides are the adjacent and the hypotenuse. To determine the measure of the angle, we can use the cosine function.
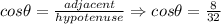
To determine the value of the angle, multiply both sides of the inverse of cosine.
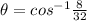
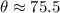
The value of the θ in Triangle 1 is approximately 75.5°.
Moving on to the next triangle, let's identify the sides with respect to the angle.
Since the given sides are the opposite side and the hypotenuse, we can use the sine function to solve for the value of the angle.
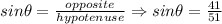
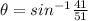
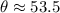
The value of θ in Triangle 2 is approximately 53.5°.
Let's now identify the given sides in the third triangle with respect to angle θ.
Since the given sides are the adjacent side and the opposite side, we can use the tangent function to solve for the value of the θ.
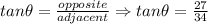
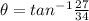
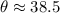
The value of θ in Triangle 3 is approximately 38.5°.
Lastly, for the 4th triangle, let's the given sides with respect to angle θ.
Similar to the third triangle, the given sides are the opposite side and the adjacent side. With this, we can use the tangent function to identify the value of θ.
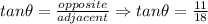
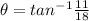
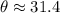
The value of θ in Triangle 4 is approximately 31.4°.
Here is the summary of the value of each θ.