From Thales's theorem, which states that in a inscribed triangle, where its hypotenuse its the diameter of a circle, its angle opposite to the hypotenuse is a right angle, so we get
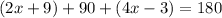
because interior angles of a triangle add up to 180 degrees. By combining similar terms, from our last relation, we get
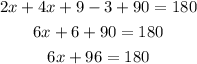
By moving 96 to the right hand side, we have
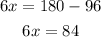
Then, x is given by
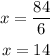
Therefore, the answer is option B.