Question

Complete the description of what happens to a figure when the given sequence of transformations isapplied to it:(x,y) → (x,y); (x,y) → (0.5x,0.5y);(x,y) → (x - 7,y + 7).Reflection over the (select)and(select)with a scale factor of 0.5; translation 7 units leftunits up.

Answer 1

box1: y-axis

box2: dilation

box3:7

Step-by-step explanation

`\begin{gathered} Let\text{ } \\ (x,y)\text{ the initial image} \end{gathered}`

Step 1

`(x,y)\rightarrow(-x,y)`

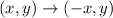
we can see y coordinate is the same, but x coordiante has opposite sign(its sign is changed)

hence

When you reflect a point across the y-axis, the y-coordinate remains the same, but the x-coordinate is transformed into its opposite (its sign is changed),so for box 1 the answer is

y-axis

Step 2

`(x,y)\rightarrow(0.5x,0.5y)`

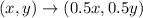
A dilation (similarity transformation) is a transformation that changes the size of a figure. It requires a center point and a scale factor , k

in this case k=0.5

`\begin{gathered} (x,y)\rightarrow(kx,ky) \\ (x,y)\rightarrow(0.5x,0.5y) \\ so \\ k=0.5 \end{gathered}`

so, for box 2, the answer is dilation

Step 3

`(x,y)\rightarrow(x-7,y+7)`

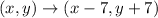
when you traslate horizontally , you affect the x coordinates, so

`\begin{gathered} x+k\rightarrow moves\text{ the xcoordiantes k espaces to the rigth} \\ x-k\rightarrow moves\text{ the xcoordiantes k espaces to the left} \end{gathered}`

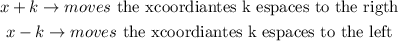
hence, in this case

`(x-7)\rightarrow the\text{ figure is traslated 7 units to the left}`

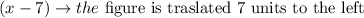
and

when you traslate the figure vertically, you move on y axis, it means

`\begin{gathered} y+k=\text{moves the shape up} \\ y-k=\text{moves the shape down} \end{gathered}`

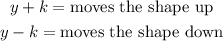
hence, in this case

`y+7\rightarrow th\text{e figure is traslated 7 units up}`

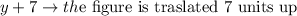
I hope this helps you