If mAB = 72 degrees, then the central angle O will have the same measure.
Now, to find the sector area of a circle, we use the next equation:
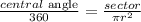
Where :
r = radius = 10
O = Central angle = 72 degrees.
Solve for the area of the sector AS:
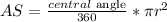
Replacing:
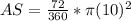
Solve it:
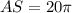
Hence, the area of the vector is 20π.