Question

A person runs with an initial velocity of 5.0m/s . Then he slows down at a constant rate of -1.2 m/s over a period of 3 second.A. What is his velocity at the end of the time?B . What distance does he travel during the whole process

Answer 1

A) the velocity at the end of the time is 1.5 m/s

B)the total distance traveled is 4.2 meters

Step-by-step explanation

Velocity is the rate at which the position changes,and acceleration is the rate of change in velocity over time

the acceleration can be calculated usign the expression:

`a=\frac{\Delta velocity\text{ }}{\Delta\text{ time}}=\frac{v_f-v_i}{\text{t}}`

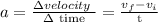
Step 1

A. What is his velocity at the end of the time?

we need to use the formula

`\begin{gathered} v_f=v_i+at \\ where \\ v_i\text{ is the initial velocity} \\ a\text{ is the acceleration } \\ t\text{ is the time} \end{gathered}`

so, replace

`\begin{gathered} v_f=v_i+at \\ v_f=5+(-1.2(m)/(s^2))(3\text{ s)} \\ v_f=(5-3.6\text{ )}\frac{m}{s^{}} \\ v_f=1.4\frac{m}{s^{}} \end{gathered}`

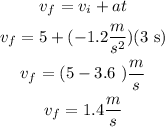
so, the velocity at the end of the time is 1.5 m/s

Step 2

What distance does he travel during the whole process :

to find this we need use the equation :

`d=v_1t+(1)/(2)at^2`

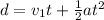
so,let

`\begin{gathered} v_i=5\text{ }(m)/(s) \\ t=\text{ 3 s} \\ a=-1.2(m)/(s^2) \end{gathered}`

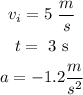
replace

`\begin{gathered} d=v_1t+(1)/(2)at^2 \\ d=(5\text{ }(m)/(s))(3\text{ s)+}(1)/(2)(-1.2(m)/(s^2))(3s)^2 \\ d=15\text{ m-}10.8\text{ m} \\ d=4.2\text{ m} \end{gathered}`

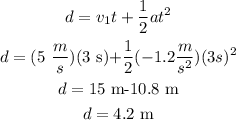
so

the total distance traveled is 4.2 meters

I hope this helps you