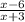Question

How would I simplify the rational expression x^2_2x-24÷ x^2+7x+12?

Answer 1

we have the expression

`(x^2-2x-24)/(x^2+7x+12)`

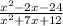
step 1

Simplify the numerator

`x^2-2x-24`

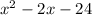
Solve the quadratic equation, using the formula

a=1

b=-2

c=-24

substitute

`x=\frac{-(-2)\pm\sqrt[]{(-2)^2-4(1)(-24)}}{2(1)}`
`\begin{gathered} x=\frac{2\pm\sqrt[]{100}}{2} \\ \\ x=(2\pm10)/(2) \end{gathered}`

the values of x are

x=6 and x=-4

therefore

`x^2-2x-24=(x+4)(x-6)`

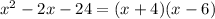
step 2

Simplify the denominator

`x^2+7x+12`

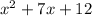
Solve the quadratic equation, using the formula

a=1

b=7

c=12

substitute

`x=\frac{-7\pm\sqrt[]{7^2-4(1)(12)}}{2(1)}`
`x=(-7\pm1)/(2)`

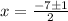
the values of x are

x=-3 and x=-4

therefore

`x^2+7x+12=(x+3)(x+4)`

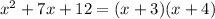
step 3

substitute the given values in the original expression

`((x+4)(x-6))/((x+3)(x+4))`

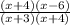
simplify

`(x-6)/(x+3)`