Question

Find the dimensions of a rectangular door that has a perimeter of 220in if the width is 50in less than the height of the door.

Answer 1

height = 80in

width = 30 in

Step-by-step explanation

The perimeter of the door is the sum of twice the width and twice the height:

`220=2w+2h`

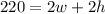
We also know that the width is 50in less than the height:

`w=h-50`

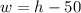
Replace w by this equation into the perimeter:

`220=2(h-50)+2h`

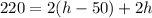
Solve for h. Apply distributive property:

`220=2h-100+2h`

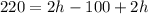
Add like terms:

`220=4h-100`

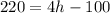
Add 100 to both sides of the equation

`\begin{gathered} 220+100=4h-100+100 \\ 320=4h \end{gathered}`

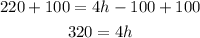
Divide both sides by 4

`\begin{gathered} (320)/(4)=(4h)/(4) \\ 80=h \end{gathered}`

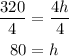
The height of the door is 80 in.

Now replace h = 80 into the equation of the width of the door:

`w=80-50=30`

The width of the door is 30 in.