We must find the area of the bigger circle and then the area of the smaller circle.
Area of the bigger circle:
Using the formula for the area, we have:
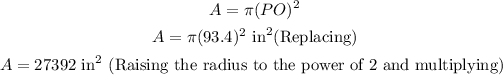
Area of the smaller circle:

Area of the shaded region:
The area of the shaded region is: Ab - As (Ab:Area of the bigger circle, As: Area of the smaller circle)
The area of the shaded region is: (27392 - 1506) square inches = 25886 square inches.
The answer is the option C.