Question

You and your buddy are scuba diving and notice that air bubbles triple in volume as they rise to the surface from where you are in the ocean. Ignoring any temperature changes, how far below the surface of the water (in m) are you when these bubbles are released? The ocean has a density of 1,031 kg/m3.

Answer 1

`h=20.06\text{ m}`

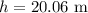
Step-by-step explanation

Since the temperature is constant, applying the ideal gas equation, we have that the product of volume and pressure remains constant:

`P_1V_1=P_2V_2`

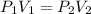
When they are submerged in water, their pressure is:

`P_1=P_(atm)+h\rho g`

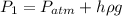
where Patm = atmospheric pressure

h = depth below the surface

ρ = density

g = acceleration due to gravity

At the surface of the water:

`P_2=P_(atm)`

Applying the ideal gas equation, we have that:

`P_1V_1=P_2V_2`

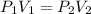
But we have that V2 = 3V1. Substituting that into the equation:

`\begin{gathered} P_1V_1=P_2(3V_1) \\ \\ \Rightarrow P_1=3P_2 \\ \\ P_(atm)+h\rho g=3P_(atm) \\ \\ h\rho g=3P_(atm)-P_(atm)=2P_(atm) \\ \\ h=(2P_(atm))/(\rho g) \end{gathered}`

Substitute the given values into the equation and solve for h:

`\begin{gathered} h=(2*101325)/(1031*9.8) \\ \\ h=20.06\text{ m} \end{gathered}`

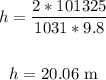
That is the height when the bubbles are released.