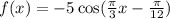Question

The graph of a cosine function shows a reflection over the x-axis, an amplitude of 5, a period of 6, and a phase shift of 0.25 to the right.Which is the equation of the function described?a. f(x)=−5cos(πx/3−1/4)b. f(x)=−5cos(πx/3 − π/12)c. f(x)=−5cos(3πx − 1/4)d. f(x)=−5cos(πx/3 − 4π/3)

Answer 1

Given that:

The graph of a cosine function shows a reflection over the x-axis, an amplitude of 5, a period of 6, and a phase shift of 0.25 to the right.

To find the equation of the function described.

Step-by-step explanation:

we have that,

General formula of cosine function is,

`f(x)=a\cos(b(x+c))+k`

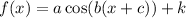
where a is amplitude, b is period factor, c is shift (left/right) and k is shift (up/down)

From given,

a=5

b=2pi/6=pi/3

c=0.25

we get,

`f(x)=-5\cos(\pi)/(3)(x-0.25)`

`f(x)=-5\cos(\pi)/(3)(x-(1)/(4))`

`f(x)=-5\cos((\pi)/(3)x-(\pi)/(12))`

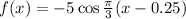
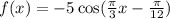
Answer is: option:b

`f(x)=-5\cos((\pi)/(3)x-(\pi)/(12))`