In order to find the area of a triangle with 3 sides, we use the Heron's formula which says if a, b, and c are the three sides of a triangle, then its area is,
![\begin{gathered} Area=A=\sqrt[]{S(S-a)(S-b)(S-c)} \\ S=\text{Semiperimeter}=(a+b+c)/(2) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/84c5p14s8yd5w3oc0g3ng8928vp985to2c.png)
Given a triangle with a = 19, b = 14, c = 19, the area is as shown below:
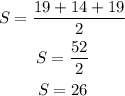
![\begin{gathered} A=\sqrt[]{S(S-a)(S-b)(S-c)} \\ A=\sqrt[]{26(26-19)(26-14)(26-19)} \\ A=\sqrt[]{26(7)(12)(7)} \\ A=\sqrt[]{15288} \\ A=123.6447 \\ A=123.6(\text{nearest tenth)} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/x99dw89joqwady76tcmdpqtei4ltq7i4dv.png)
Hence, the area of the triangle is 123.6 square unit correct to the nearest tenth