Question

Applications Involving Rational Equations Create an equation that represents each scenario. Then use that equation to solve the given problem.

Answer 1

Miriam needs 8 consecutive throws to win

Here, we want to calculate the number of throws consecutively that Miriam must make into the cup to beat Ishak

Since Ishak made 48% already, Miriam must make 52%

Out of 30, she made 12;

This represents a percentage of;

`(12)/(30)\text{ }*\text{ 100\% = 40\%}`

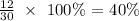
Let the number of throws needed be x;

So;

`\begin{gathered} (x+12)/(30+x)\text{ }*\text{ 100 = 52} \\ \\ 100(x+12)\text{ = 52(30+x)} \\ \\ 100x\text{ + 1200 = 1560+52x} \\ \\ 100x-52x\text{ = 1560-1200} \\ \\ 48x\text{ = 360} \\ \\ x\text{ = }(360)/(48) \\ \\ x\text{ = 7.5 which is approx 8} \end{gathered}`

So what this mean is that, out of 38 throws, 20 would enter, with the last 8 being consecutive