Question

y=3x+5y=ax+bwhat value for a and b make the system inconsistent? what values for a and b the system consistent and dependent? Explain

Answer 1

A system of equation is said to be consistent if it has at least one solution.

If a consistent system has an infinite number of solutions, it is dependent.

Given the system of the equation:

`\begin{gathered} y=3x+5 \\ y=ax+b \end{gathered}`

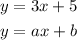
For the system to be dependent and consistent, they must be the same line.

Therefore, the values for a and b that make the system consistent and dependent are:

a=3, b=5

A system is said to be inconsistent if it has no solution. That is, the two lines do not intersect (are parallel).

Therefore, the values of a and b that make the system inconsistent are:

a=3, b= -5