From the problem, we have the inequality :

Substract both sides by 3
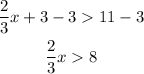
Cross multiplication :

Divide both sides by 2 :
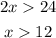
The solution is x > 12
To grahp this inequality, take note that the graph will be composed of a circle or endpoint and an arrow
If the inequality sign is < or >, the endpoint is an open circle.
If the inequality sign is ≤ or ≥, the endpoint is a closed or shaded circle.
Since the inequality symbol in the question is >, we will use an open circle.
The graph will look like this.
The endpoint is located at x = 12, and the direction is to the right since the sign is greater than ">"