Given:
a.) An equation that passes through the point (6, -2).
b.) A slope of -4.
Recall, the slope-intercept form:

Where,
m = slope
b = y - intercept
x, y = coordinates of the point that pass through the graph
a.) Let's first determine the y-intercept (b). Substitute x, y = 6, -2 and m = -4 in y = mx + b.

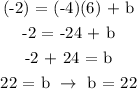
Therefore, b = 22
b.) Let's now complete the equation. Substitute m = -4 and b = 22 in y = mx + b.

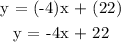
Therefore, the equation of the line is y = -4x + 22