Step-by-step explanation
Step 1
set the equations:
Let x represents the cost of 1 pen
Let y represents the cost of 1 copybook
Let z represents the cost of 1 ruler
so
a)2 pens and 1 copybook cost $16.50
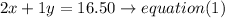
b)4 pens and 5 copybooks cost $52.50

c) 2 copy books and 3 rulers and paid $41
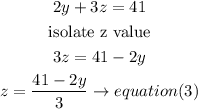
Step 2
solve the equations:
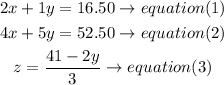
a) isolate the x value in equation (1) and (2) then set equal each other,
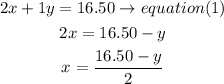
and
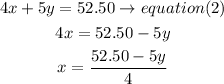
so
![undefined]()
c