(a) A graph of the relationship between the cost of transportation in dollars C(x) and mileage x over the entire 960-mile route is graph D.
(b) The cost as a function of mileage for hauls between 100 and 400 miles from the first city is C(x) = 0.47x + 8.
(c) The cost as a function of mileage for hauls between 400 and 800 miles from the first city is C(x) = 0.3x + 55.
Part a.
Since the company charges, for each pound, $0.55 per mile for the first 100 miles, the rate of change (slope) is given by;
slope (m) = 55/100 = $0.55 per mile.
Total cost, C(x) = 0.55 × 100 = $55 ⇒ (100, 55)
For the next 300 miles, the slope is given by;
slope = $0.40 per mile
Total cost, C(x) = (0.55 + 3(0.40)) × 100 = $175.
Total cost, C(x) = $175 ⇒ (400, 175)
For the next 400 miles, the slope is given by;
slope = $0.30 per mile
Total cost, C(x) = (1.75 + 4(0.30)) × 100 = $295.
Total cost, C(x) = $295 ⇒ (800, 295)
For the remaining 160 miles, there is no charge, which means the total cost would be $2.95 ⇒ (960, 175).
Based on the calculations, only graph D correctly models the relationship.
Part b.
For hauls between 100 and 400 miles from the first city, the total cost can be calculated by using these points (100, 55) and (400, 195) as follows;
Slope (m) = (195 - 55)/(400 - 100)
Slope (m) = $0.47 per mile.
At data point (100, 55) and a slope of 0.47, a linear equation for the total cost can be calculated by using the point-slope form as follows:
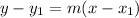
y - 55 = 0.47(x - 100)
C(x) = 0.47x - 47 + 55
C(x) = 0.47x + 8
Part c.
For hauls between 400 and 800 miles from the first city, the total cost can be calculated by using these points (400, 175) and (800, 295) as follows;
Slope (m) = (295 - 175)/(800 - 400)
Slope (m) = $0.3 per mile.
At data point (400, 175) and a slope of 0.3, a linear equation for the total cost can be calculated by using the point-slope form as follows:
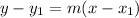
y - 175 = 0.3(x - 400)
C(x) = 0.3x - 120 + 175
C(x) = 0.3x + 55