Question

Graph the line with the equation y = 4/3 x - 4

Answer 1

we are given the equation of a line, and we are asked to find the graph. To do that, let's remember that in order to find the graph of a line, we must find two points that lie on that line. The first number can be the y-intercept, let's remember the general equation for a line:

`y=mx+b`

where "m" represents the slope and "b" the intercept. In this case, the equation is:

`y=(4)/(3)x-4`

In this case, the intercept is b = -4. So our first point is (0,-4)

the second point can be found by making y = 0 and solving for "x", like this:

`\begin{gathered} y=(4)/(3)x-4 \\ 0=(4)/(3)x-4 \end{gathered}`

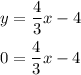
Adding 4 on both sides

`4=(4)/(3)x`

multiplying both sides by 3/4

`\begin{gathered} 4((3)/(4))=x \\ x=3 \end{gathered}`

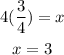
So the second point is (3,0). Now we locate the points and join them using a line, since the slope is a positive number (m=4/3) the line should go upwards. The graph is like this: