The formula for any give rectangle is just that you multiply it's lenght by it's width, or as expressed by the equation;
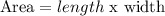
Since in the picture we are given a side of the rectangle measuring 4 inches, with it's length as unkown, we can only assume that the 4 inches there is the width therefore for a sqaure with an area of 34 square inches, we can express it as;

Therefore the lenght of the rectangle in our question is 8.5 inches.