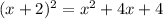To identify the error in the procedure shown in the picture, it is important to remember the following:
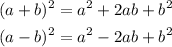
Keeping this on mind, you can solve the given equation as following:
1. Given:
![\sqrt[]{5x}=x+2](https://img.qammunity.org/2023/formulas/mathematics/college/a0ce88gr7tap8jdw4o0zexezw4cwl5qwnc.png)
2. Square both sides of the equation:
![\begin{gathered} (\sqrt[]{5x})^2=(x+2)^2 \\ 5x=x^2+2(x)(2)+2^2 \\ 5x=x^2+4x+4 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/urqxgjl9k5wyhrkmtci3b7p4kdz0nbbo2k.png)
3. Move the the term on the left side to the right side and add like terms:
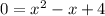
4. Apply the Quadratic formula to find "x":
![\begin{gathered} x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ \\ x=\frac{-(-1)\pm\sqrt[]{(-1)^2-4(1)(4)}}{2(1)} \\ \\ x_1=(1)/(2)(i-\sqrt[]{15)} \\ \\ x_2=(1)/(2)(i+\sqrt[]{15)}_{} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/udd06ghsqiguac0uq87klazgc4ymi2my4f.png)
Therefore, you can identify that the error is in Step C, because: