Question

Trudy Aden deposited $1350 in a new credit union savings account on the first of the quarter. The principle earns 3.56% interest compounded quarterly. She made no other deposits or withdrawls. A. what is the compound interest?B. what was the amount in her account at the end of 6 months?C. what is the compound interest?D. what was her account's amount at thr end of one year?

Answer 1

We have a principal which compound its interest quarterly.

The annual nominal rate is 3.56%.

The principal is $1350.

We can express the amount in the account with the expression:

`FV=PV\cdot(1+(r)/(m))^(n\cdot m)`

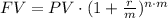
where FV: future value, PV: present value, r: annual interest rate, n: number of years, m: number of subperiods a year.

In this problem, r=0.0356, PV=1350 and m=12/3=4

In this case, for a period of 6 months, we have n=0.5, so the calculation gives a final vlaue of:

`FV=1350\cdot(1+(0.0356)/(4))^(0.5\cdot4)=1350\cdot1.0089^2\approx1350\cdot1.018\approx1374.14`

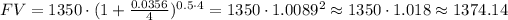
Substracting the principal of 1350, the interest is:

`I=1374.14-1350=24.14`

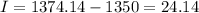
For a one-year period, n=1, so we can calculate the final value as:

`FV=1350\cdot(1+(0.0356)/(4))^(1\cdot4)=1350\cdot1.0089^4\approx1350\cdot1.0361\approx1398.71`

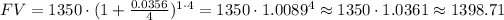
Again, by substracting the principal, we can get the compounded interest:

`I=1398.71-1350=48.71`

A. For a period of 6 months, the interest is $24.14.

B. The balance at 6 months from the deposit is $1374.14.

C. For a period of one-year, the interest is $48.71.

D. The balance at one year from the deposit is $1398.71.