We need to find the value of t for which h(t) = 0.
Notice that a quadratic equation may have two real solutions. Since t starts at 0 and only grows, we are interested in the positive solution only.
We have:
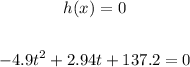
Then, using the quadratic equation, we obtain:
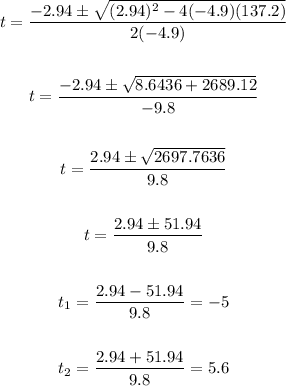
Answer
The object strikes the ground at: t = 5.6 seconds.