Question

Ball A, initially moving at 4.6 m-s-1, strikes a stationary ball B with the same mass.After colliding, ball A moves with a speed of 2.4 m-s-1 at an angle of 16° above theoriginal line of motion, while ball B moves with speed u at an angle of theta below theoriginal line of motion.A) Calculate the angle Theta (in °) below the original line of motion that ball B moves afterthe collisionB) Calculate the speed u (in m-s-1) of ball B after the collision.

Answer 1

A)

Let:

u1 = Initial speed of ball A = 4.6

v1 = Initial speed of ball B = 0

u2 = Final speed of ball A = 2.4

v2 = Final speed of ball B = u

m1 = m2 = Mass of ball A

So:

Using the conservation of momentum:

`m1u1+m2v1=m1u2+m2v2`

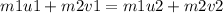
Since the masses are the same:

`\begin{gathered} u1+v1=u2+v2 \\ \\ \end{gathered}`

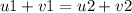
Expressing the speeds in a rectangular forms:

`\begin{gathered} u1=4.6i \\ u2=2.4\cos (16)+2.4\sin (16)=2.31i+0.66j \\ v2=u=u_x+u_y \end{gathered}`

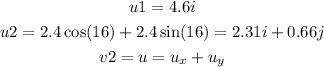
So:

`4.6i=2.31i+0.66j+v_xi+v_yj_{}`

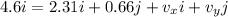
So:

`\begin{gathered} 4.6i=2.31i+v_xi \\ 0=0.66j+v_yj \\ So\colon \\ v_xi=4.6i-2.31i=2.29i \\ v_yj=-0.66j \end{gathered}`

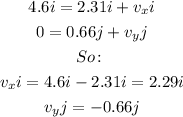
Now, we can calculate the angle as follows:

`\begin{gathered} \theta=\tan ^(-1)((v_yj)/(v_xi)) \\ \theta=\tan ^(-1)((-0.66)/(2.29)) \\ \theta=-16.08^(\circ) \end{gathered}`

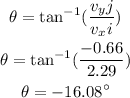
B) The speed is given by:

`\begin{gathered} u=\sqrt[]{v_xi^2+v_yj^2} \\ u=\sqrt[]{2.29^2+(-0.66)^2} \\ u=2.38(m)/(s) \end{gathered}`