Question

Find the slant height of the cone need homework help

Answer 1

Slant Height of a Cone

We are given a cone where the height BC = 6 in and the diameter is 4 in.

The radius is half the diameter, thus AB = 4/2 = 2 in

It's required to find the slant height of the cone, i.e., the value of AC.

Note ABC is a right triangle where the right angle is at vertex B. Thus the hypotenuse is AC and the legs are AB and BC.

Applying the Pythagorean's Theorem:

`AC^2=AB^2+BC^2`

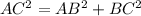
Substituting values:

`\begin{gathered} AC^2=2^2+6^2 \\ \text{Calculating:} \\ AC^2=4+36 \\ AC^2=40 \end{gathered}`

Taking the square root:

`\begin{gathered} AC=\sqrt[]{40} \\ AC=\sqrt[]{4\cdot10} \\ AC=\sqrt[]{4}\cdot\sqrt[]{10} \\ AC=2\text{ }\sqrt[]{10} \end{gathered}`