Given the function:
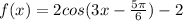
You need to remember that the General Equation of the Cosine Function is:

Where:
- The Amplitude is:
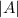
- The Period is:

- The horizontal shift is "C".
- The Vertical Shift is "D".
- The midlline is:
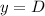
In this case, you can identify that:
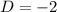
Therefore, the midline is: