ANSWER:
(a)
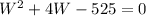
(b)
Width: 21 feet
Length: 25 feet
Explanation:
Given:
Width (W) = W ft
Length (L) = (4 + W) ft
Area (A) = 525 ft^2
(a)
The area of a rectangle is equal to the product of its length and width, therefore:
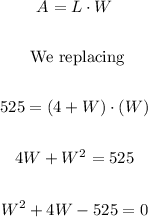
(b)
We solve the equation by factoring:
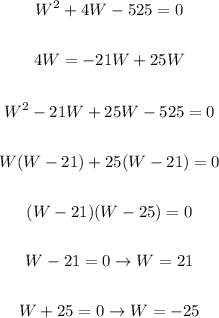
The width of the rectangle is equal to 21 feet and the length of the rectangle is equal to 25 feet.