Question

If 2x + 5 = 8x, then 12x = ?A.5B.10C.15D.20

Answer 1

`10.\text{ Option B is correct}`

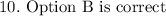
Step-by-step explanation:

Given the equation expressed as:

`2x+5=8x`

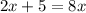
First, we need to calculate the value of "x" from the given expression.

Step 1: Given the equation 2x + 5 = 8x

Step 2: Subtract 5 from both sides:

`\begin{gathered} 2x+5-5=8x-5 \\ 2x+\cancel{5}-\cancel{5}=8x-5 \\ 2x=8x-5 \end{gathered}`

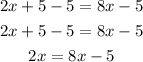
Step 3: Subtract 8x from both sides

`\begin{gathered} 2x-8x=8x-8x-5 \\ -6x=\cancel{8x}-\cancel{8x}-5 \\ -6x=-5 \end{gathered}`

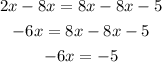
Step 4: Divide both sides by -6

`\begin{gathered} (-6x)/(-6)=(-5)/(-6) \\ x=(5)/(6) \end{gathered}`

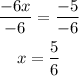
Step 5: Get the value of 12x. Substitute x = 5/6 into the expression to have:

`\begin{gathered} 12x=12((5)/(6)) \\ 12x=\cancel{12}^2(\frac{5}{\cancel{6}^1}) \\ 12x=2*5 \\ 12x=10 \end{gathered}`

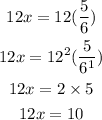
Therefore the value of 12x is 10