Question

Drag each volume of a prism to match a rectangular prism on the left with the given dimensions.54 cu. in.72 cu. in.36 cu. in.80 cu. in.Dimensions of Rectangular PrismVolume of Rectangular Prism 4 in., 3 in., 6 in. 6 in., 3 in., 2 in. 4 in., 5 in., 4 in. 2 in., 9 in., 3 in.

Answer 1

We need to determine the volume of each of the given rectangular prisms.

We know that the volume V of a rectangular prism with dimensions a, b, and c is given by:

`V=a\cdot b\cdot c`

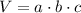
Thus, to find the volume of each rectangular prism, we need to multiply its dimensions.

We obtain:

`\begin{gathered} 4in,3in,6in\Rightarrow V=4in\cdot3in\cdot6in=(4\cdot3\cdot6)(in\cdot in\cdot in)=72in^(3) \\ \\ 6in,3in,2in\Rightarrow V=6in\cdot3in\cdot2in=(6\cdot3\cdot2)in^(3)=36in^(3) \\ \\ 4in,5in,4in\Rightarrow V=4in\cdot5in\cdot4in=(4\cdot5\cdot4)_{}in^(3)=80in^(3) \\ \\ 2in,9in,3in\Rightarrow V=2in\cdot9in\cdot3in=(2\cdot9\cdot3)in^(3)=54in^(3) \end{gathered}`

Therefore, dragging each volume to its respective place, we obtain: