Step-by-step explanation:
Given;
We are given the graph of a parabola along with the following details;
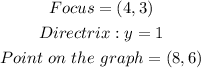
Required;
We are required to determine which statement(s) is/are correct among the options provided.
Solution;
To find the equation of a parabola given its focus and directrix, we shall take note that the parabola contains the set of points equidistant from the focus and the directrix.
We are given the following information;
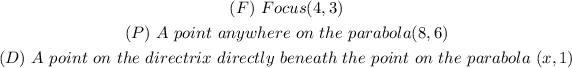
Note that the distance from F to P must equal the distance from D to P.
We can now find the distances between both FP and DP using the distance formula;
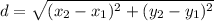
Take the point on the parabola as point (x, y);
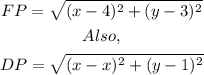
Since they are equidistant, we simply equate both formulas;

Square both sides and then simplify;
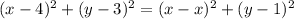
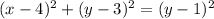
To determine the answer to option C, we shall substitute the values (8, 6) for (x, y) into the distance formula above;
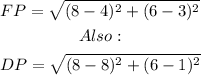
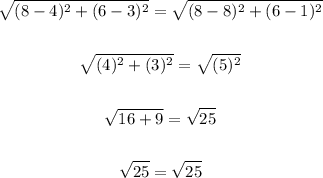
Therefore option C is correct.
The correct options so far are
Option B
Option C
Option D
Using the x value of 0.5, if we inserted this into the equation as derived above, the value of y would be, y = 5.063. That is;

Therefore the points (0.5, 5) is NOT on the parabola.
Also option F is NOT correct.
ANSWER:
The correct options based on the information available are B, C and D