Given the word problem, we can deduce the following information:
Volume of a rectangular pyramid=64 units^3
length of the rectangular base = 4 units
width of the rectangular base = 6 units
To determine the height of the pyramid, we use the formula as shown below:
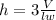
where:
V= Volume of the rectangular pyramid
l=length of the rectangular base
w=width of the rectangular base
h=height of the pyramid
We plug in what we know:

Therefore, the height of the pyramid is 8 units.