Question

here is the continuity checklist to use1.The function is defined at x = a; that is, f(a) equals a real number.2.The limit of the function as x approaches a exists.3.The limit of the function as x approaches a is equal to the function value at x = a.

Answer 1

Notice that

Therefore, f(-5) is not a real number.

2) Calculating the limit when x->a from the left and right,

`\lim_(x\to-5^+)f(x)=\lim_(x\to-5^+)(2x^2+3x+1)/(x^2+5x)=\lim_(x\to-5^+)(2x^2+3x+1)/(x(x+5))\approx(36)/(-5(\epsilon))\approx(36)/(-5\epsilon)\rightarrow-\infty`

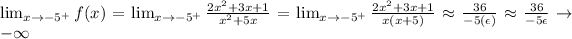
Because x^2+5x=x(x+5), and approaching from the right (x+5)>0; therefore, in the limit f(x)->-infinite

ϵ is a small positive number that approaches zero as a->-5

Similarly, in the case of approaching x->-5 from the left,

`\lim_(x\to-5^-)f(x)\approx(36)/(-5(-\epsilon))\approx\infty`

Therefore, since the limits approaching x->-5 from the left and right are not the same,

the limit of f(x) when x->-5 does not exist.

The function is not continuous at x=a and, furthermore, the limit of f(x) when x->-5 does not exist.