Given,
The combined mass of the sledge and the younger sister, m=35 kg
The angle made by the rope, θ=13°
The force applied, F=270 N
The coefficient of friction, μ=0.27
The acceleration due to gravity, g=9.8 m/s²
(a) The normal force is given by,
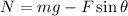
On substituting the known values,
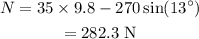
Thus the normal force is given by 282.3 N
(b) The net force on the sledge is
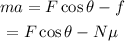
Where f is the frictional force.
Thus the acceleration will be,
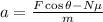
On substituting the known values,
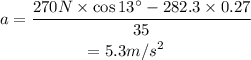
Thus the acceleration of the sledge is 5.3 m/s²