We can graph the situation as follows:
Since the shadow must have the same angle, we can use the tangent of this angle to find the height of the tower.
Then, we have that:
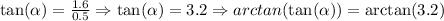
Then
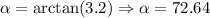
Thus, we can use this angle to find the height of the Eiffel Tower:

Then, the height of the Eiffel Tower, according to this, is 300 m.