Question

Find the solution of the system of equations. 4x - y = 11 2.r – 4y = –26

Answer 1

Step-by-step explanation:

We can use the substitution method to solve this system.

First we clear y from the first equation:

`\begin{gathered} 4x-y=11 \\ 4x-11=y \\ y=4x-11 \end{gathered}`

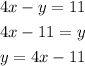
Then we replace this expression into the second equation:

`\begin{gathered} 2x-4y=-26 \\ 2x-4(4x-11)=-26 \end{gathered}`

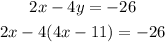
Solve for x:

`\begin{gathered} 2x-16x+44=-26 \\ -14x=-26-44 \\ x=(-26-44)/(-14)=(-70)/(-14)=5 \end{gathered}`

And with x = 5 we replace it into the first equation and solve for y:

`y=4x-11=4\cdot5-11=20-11=9`

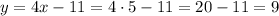
Answer:

The solution is (5, 9)