Question

Perform the indicated operation to determine if the givensimplification is correct. If it is correct, select TRUE. If it is notcorrect, select FALSE.

Answer 1

Step 1:

In this question, we are given the following:

Perform the indicated operation to determine if the given simplification is correct.

If it is correct, select TRUE. If it is not correct, select FALSE.

Step 2:

The details of the solution are as follows:

`\frac{x+2}{x^2+\text{ 5 x + 6 }}\text{ . }\frac{x+\text{ 3}}{x+7}`



`\frac{x+\text{ 2}}{(x+\text{ 3) ( x+ 2) }}.\text{ }\frac{x+\text{ 3}}{x+\text{ 7}}`
`\frac{(x+2)(x+\text{ 3)}}{(x+3)(x+2)(x+7)}=\text{ }(1)/((x+7))_{}`

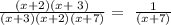
CONCLUSION:

The simplification is CORRECT.

Hence, we select TRUE