Question

Determine the measure of

Answer 1

We will use the cosine rule to find the measure of angle Q

The cosine rule is

`(RS)^2=(QR)^2+(QS)^2-2(QR)(QS)\cos \angle Q`

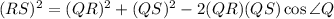
RS = 15, QR = 13, QS = 12

Substitute them in the rule above

`\begin{gathered} (15)^2=(13)^2+(12)^2-2(13)(12)\cos \angle Q \\ 225=169+144-312\cos \angle Q \\ 225=313-312\cos \angle Q \end{gathered}`

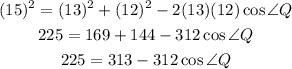
Subtract 313 from both sides

`-88=-312\cos \angle Q`

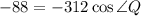
Divide both sides by - 312

`\begin{gathered} (-88)/(-312)=\cos \angle Q \\ (11)/(39)=\cos \angle Q \end{gathered}`

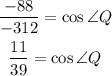
Now we will find the value of the angle Q

`m\angle Q=\cos ^(-1)(11)/(39)=73.61733`

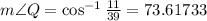
Round it to the nearest tenth

The measure of angle Q is 73.6 degrees

First answer

You can consider it as 73.5 degrees