Question

Hi. Been out due to medical reasons trying to catch up. thanks in advance

Answer 1

We have a mixture of 300 ml with 15% alcohol content, this means that in this mixture we have an alcohol total of:

`\text{alcohol}=300\cdot(15)/(100)=45\text{ ml}`

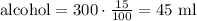
And we want to obtain a 60% alcohol solution by mixing a 90% mixture with the 15% one we have.

`\begin{gathered} \text{volume 90 \%}=v \\ \text{ alcohool content}=0.9\cdot v \end{gathered}`

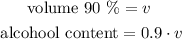
When we add this volume to what we already have, we will obtain:

`\begin{gathered} \text{ final mixture}=300+v \\ \text{alcohool content}=45+0.9\cdot v \end{gathered}`

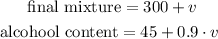
And what we want is that the alcohol content must be equal to 60% of the final mixture, so we have:

`\begin{gathered} 60\text{ \%}\cdot\text{ final mixture}=\text{ alcohol content} \\ (60)/(100)\cdot(300+v)=45+0.9\cdot v \\ 0.6\cdot(300+v)=45+0.9\cdot v \\ 180+0.6\cdot v=45+0.9\cdot v \\ \text{0}.9\cdot v-0.6\cdot v=180-45 \\ 0.3\cdot v=135 \\ v=(135)/(0.3)=450\text{ ml} \end{gathered}`

This means that we need to add 450 ml of the 90% solution to obtain the desired final mixture.