The system of inequalities is
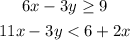
we will substitute the coordinates of each given points to find which one is the solution
∵ The 1st answer is (-4, -12)
∴ x = -4 and y = -12
We will substitute x by -4 and y by -12 and see if the inequality is right or wrong
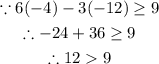

Both inequalities are right, then the answer is A