Answer:
![\begin{gathered} (A)\Rightarrow\text{ Point B lies inside the circle Because:} \\ CA=\sqrt[]{34} \\ CB=4 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/v3oal8pe95v16x8z8kpbbb7e5ye04iljp7.png)
Step-by-step explanation: The general form of the equation of the circle is as follows:
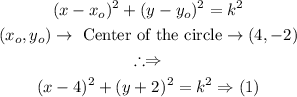
(1) is the equation of the circle. since it passes through the point (1,3), therefore substituting the (x,y) values of it in (1) gives the value complete equation (1) as follows:

(2) is the complete equation for the circle, the following graph shows if point B is inside or outside the circle.