We are asked to find the total surface area of the right triangular prism.
Recall that the total surface area of a right triangular prism is given by
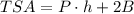
Where P is the perimeter of the base, h is the height of the prism, and B is the area of the base.
From the figure, we see that
height = 11
side 1 = 9
side 2 = 12
We can apply the Pythagorean theorem to find the length of the third side. (hypotenuse)
![\begin{gathered} c^2=a^2+b^2 \\ c^2=9^2+12^2 \\ c^2=81^{}+144 \\ c^2=225 \\ c=\sqrt[]{225} \\ c=15 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/high-school/tb8c8nfqqd8w3zcx2erx3ii959z68y8i7l.png)
Now we can find the perimeter of the base,
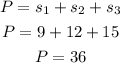
The area of the base is given by

So, the total surface area of the right triangular prism is
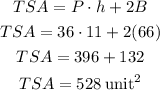
Therefore, the total surface area of the right triangular prism is 528 square units.