If (2.5) and (5,5) are the two end points of the circle, then the center of the circle is the midpoint
To find the midpoint, we will use the formula
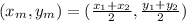
x₁ = 2 y₁=5
x₂=5 y₂=5
Substitute into the formula and evaluate
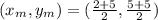
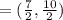
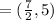
Hence, the center of the circle is (7/2 , 5)
To find the radius, we can simply find the distance between the end points and divide by 2
Using the distance formula,
![|d|=\sqrt[]{(5-2)^2+(5-5)^2}](https://img.qammunity.org/2023/formulas/mathematics/college/80ye60c8tqvaxukj5fnzq597kho1u1bxmq.png)
![=\sqrt[]{3^2}=3](https://img.qammunity.org/2023/formulas/mathematics/college/lh7ff91nfd8w0ndbnyy2vpqy0i4v66ad6i.png)
Hence, the radius is 3
The general formula for the equation of a circle centered (h,k) and radius r is given by

substitute the values into the formula
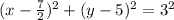
Therefore, the equation of the circle is:
