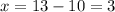Consider the following trapezoid
The formula to find the area of this trapezoid is given by the formula
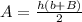
In this case, we have h=8, b=x and B=10. So the formula for our trapezoid is given by
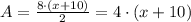
We are told that the area should be 52, so we end up with the following equation
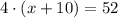
now, we should solve this equation for x. To do so, we start by dividing by 4 on both sides. So we get

Now, we subtract 10 on both sides, so we get