To find the equation of a line that passes through a point and parallel to another line, we can use the point-slope form.

First, let's find out what the slope m should be. Remember that parallel lines have the same slope.
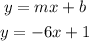
We see that the slope is -6.
Now that we have the slope, we just need to plug in the coordiantes of the point and the slope to the point-slope format of the equation. Then, we will rewrite it in point-intercept form.
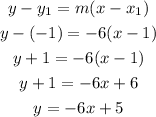
The answer is y = -6x + 5.